
© 2014 Foundation Supportworks
®
,
Inc.
All Rights Reserved
p 126
APPENDIX 2D
PILE BUCKING CONSIDERATIONS
Chapter 2
Helical Foundation Systems
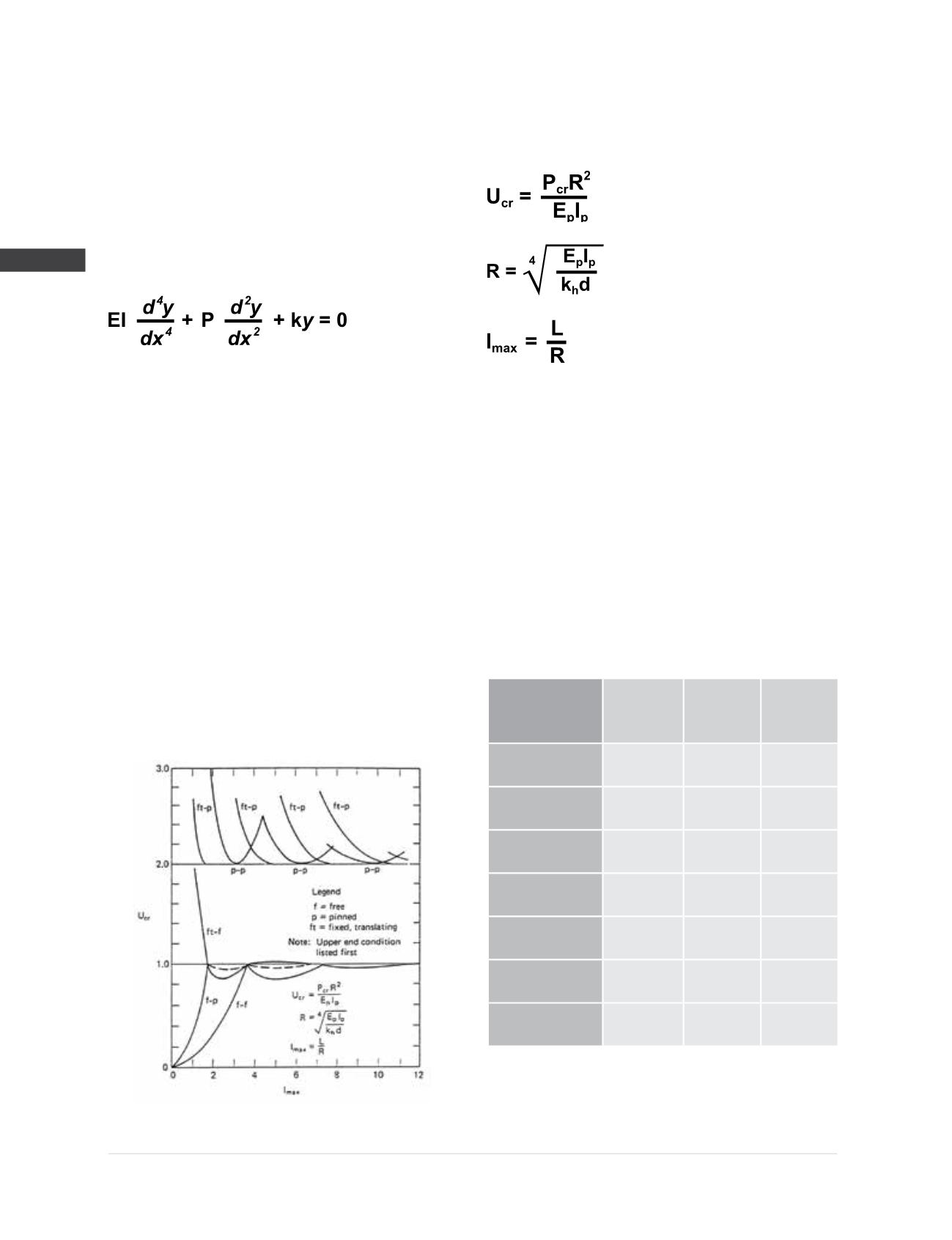
Davisson Method
The Davisson Method (1963) considers lateral
support from the surrounding soil and variable
boundary conditions for the pile. This method is
based on manipulation of the governing
differential equation which assumes the
subgrade modulus of the soil is constant with
depth along the pile:
Where,
EI
= Flexural Stiffness of the Pile
P
= Axial Load
k
= Subgrade Modulus
The differential equation was solved for various
boundary conditions using non-dimensional
variables. The boundary conditions are free (f),
pinned (p) and fixed-translating (ft). For initial
conditions where the pile is fully-embedded,
initially straight and the axial load is assumed
constant (no skin friction), the dimensionless
solutions are shown in
Figure 2D.1
. For further
discussion of the derivation of these solutions
the reader is advised to see the paper by
Davisson (1963).
The dimensionless variables are the critical axial
load coefficient (U
cr
) and the maximum value of
the depth coefficient ( I
max
) and are defined as:
Where,
P
cr
= Critical Axial Load
R
= Relative Stiffness Factor
E
p
I
p
= Flexural Stiffness of the Pile
k
h
= Horizontal Subgrade Modulus
d
= Pile Diameter
L
= Shaft Length over which k
h
is constant
Typical values of k
h
for design purposes are
shown in
Figure 2D.2
.
Soil Type-
Consistency
Cohesion
(psf)
SPT
N-value
(bpf)
Design kh
(pci)
Clay-Very Soft
<250
0-1
<30
Clay-Soft
250-500
2-4
30
Clay-Medium Stiff
500-1000
5-8
100
Sand-Very Loose
(above GWT)
NA
0-4
<25
Sand-Very Loose
(below GWT)
NA
0-4
<20
Sand-Loose
(above GWT)
NA
5-10
25
Sand-Loose
(below GWT)
NA
5-10
20
Figure 2D.2
Typical design values for horizontal subgrade modulus
(Reese, Wang et al. 2004b)
Figure 2D.1
Buckling load (load coefficient) vs. length
(depth coefficient) for k
h
= constant (Davisson 1963)












